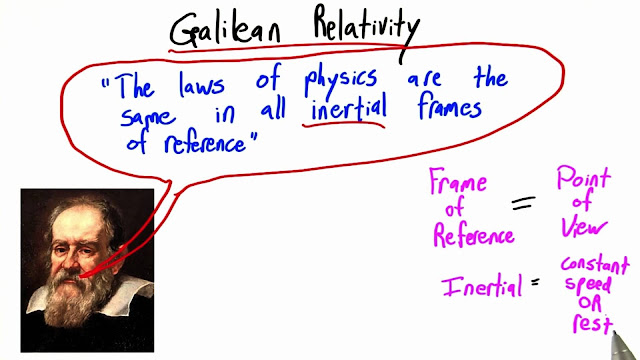
Galilean Relativity
<
RELATIVITY AND COMMON SENSE
A child walks along the floor of a moving train. Passengers on the train measure the child's speed and find it to be 1 meter per second. When ground-based observers measure the speed of the same child, they obtain a different value; observers on an airplane flying overhead obtain still another. Each set of observers obtains a different value when measuring the same physical quantity. Finding the relation between those values is a typical problem in relativity.
According to Einstein, common sense is "that layer of prejudice laid down in
the mind prior to the age of eighteen."
There is nothing at all startling about these observations; relativity was not invented by Albert Einstein. Einstein's work did, however, drastically change the way such phenomena are understood; the term "relativity" as used today generally refers to Einstein's theory.
The study of relativity began with the work of Galileo GaHlei around 1630; Isaac Newton also made important contributions. The ideas described in this chapter, universally accepted until 1900, are known as "Galilean relativity."
Galilean relativity is fully consistent with the intuitive notions that we call common sense." 1 In the example above, if the train moves at 30 meters per second (m/sec) in the same direction as the child, common sense suggests that ground-based observers should find the child's speed to be 31 m/sec; Galilean relativity gives precisely that value. Einstein's theory, as we shall see, gives a different result.
In the case of the child, the difference between the two theories is minute. The speed measured by ground observers according to Einstein's relativity differs from the Galilean value 31 m/sec only in the fourteenth decimal place; no measurement could possibly detect such a tiny difference. This result is characteristic of Einsteinian relativity: its predictions are indistinguishable from those of Galilean relativity whenever the observers, as well as all objects under observation, move slowly relative to one another. That realm is generally called the nonrelativistic limit, although Galilean or Newtonian limit would be a more apt designation. "Slowly" here means at a speed much less than the speed of light.
The speed of light plays a central role in Einstein's theory; whenever any speed in the problem approaches that value, Einsteinian relativity departs dramatically from that of Galileo and Newton. Because the speed of light is so great, however, most commonly observed phenomena are adequately described by Galilean relativity.
The special" theory of relativity, which is the principal subject of this book, is restricted to observers who move uniformly, that is, at constant speed in the same direction. If observers move with changing speeds, or along curved paths, the problem of relating their measurements is much more complicated. Einstein addressed that problem as well, in his general" theory of relativity. Because the general theory involves quite advanced mathematics. The special theory, in contrast, requires only elementary algebra and geometry and can be presented with full rigor.
Many of the conclusions of special relativity run counter to our intuition concerning the nature of space and time. Before Einstein, no one doubted that time is absolute. Newton put it as follows in his Principia: "Absolute, true, and mathematical time, of itself and from its own nature, flows equably without relation to anything external."
Special relativity obliges us to abandon the absolute nature of time. We shall see, for example, that the time order of two events can depend on the relative motion of the observers who view them. One set of observers may find that a certain event A occurred before another event B, whereas according to a second set of observers, who are moving relative to the first, B occurred before A. This result is surely difficult to accept.
In some cases, a reversal of time ordering would be truly bizarre. Suppose that at event A a moth lands on the windshield of a moving car; the car clock reads 12:00. At event B another moth lands; the car clock now reads 12:05. For the driver of the car, the order of those events is a direct sensory experience: she can see both events happen right in front of her and can assert with confidence that A happened first. If observers on the ground were to claim that event B happened first, they would be denying that sensory experience; moreover, the car clock would according to them be running backward! (It would read 12:05 before it reads 12:00.)
As we shall see, special relativity implies that moving clocks run slow. That is itself a strange result, but clocks running backward would be too much to swallow. No such disaster arises, however. In the case of the moths, event A happens first according to all observers. A reversal of time ordering can occur only for events spaced so far apart that no single observer (and no single clock) can be present at both. The order of such events is not a direct sensory experience for anyone; it can be determined only by comparing the readings of two distinct clocks, one present at event A and the other present at B. If two sets of observers disagree on the order of those events, no one's sensory experience is contradicted and no one sees any clock running backward. The proof of this assertion, depends on the fact that nothing can travel faster than light, one of the important consequences of special relativity.
A logical requirement of any theory is causality. If event A is the cause of event B, A must occur before B: the cause must precede the effect. We will see in chapter 5 that special relativity is consistent with the causality requirement. Whenever a cause-and-effect relation exists between two events, their time order is absolute: all observers agree on which one happened first.
Figure 1.1 shows a hypothetical experiment to illustrate the relativistic reversal of time ordering. Event A takes place in San Francisco and event B in New York. According to clocks at rest at those locations, A occurs before B. The same events are monitored by observers on spaceships moving from west to east at equal speeds; one ship is over San Francisco when event A occurs, and the other is over New York when event B occurs. Special relativity predicts that if the ships are moving fast enough, their clocks can show event B happening before A. Notice that no single clock is present at both events; the relevant times in the problem are recorded by four distinct clocks, two on the ground and two on the spaceships.
I hasten to add that no such experiment has ever been performed. The fastest available rockets travel a few kilometers per second, only about one hundred thousandth the speed of light. At that speed, the events of figure 1.1 would have to be separated in time by less than a millionth of a second
if a reversal of time order were to be detectable. Moreover, the speeds of the two spaceships would have to be equal to within a very small tolerance. The experiment is just too hard to carry out. But we can be confident that if faster rockets were available and if other technical requirements were met, the effect could be detected.
Fig. 1.1. Hypothetical experiment to demonstrate the reversal of time ordering predicted by special relativity. Event A occurs in San Francisco, event B in New York. Each event is detected by two sets of observers-one set fixed on earth and the other located on spaceships flying at equal (constant) speeds. Each set of observers measures the times of the two events on its own clocks, which have been previously synchronized. According to earth clocks, event A happens before B, whereas according to spaceship clocks, B happens before A. The time intervals shown on the clocks are much exaggerated.
The evidence that confirms special relativity comes principally from atomic and subatomic physics. In many experiments particles move at speeds close to that of light, and the effects of special relativity are dramatic. Particles are created and annihilated in accord with the famous Einstein relation E = mc^2 . No understanding of such phenomena, or of the kinematics of high-energy particle reactions, would be possible without relativity. Thus Einstein's theory is confirmed daily in every high-energy physics laboratory. Particle reactions are not within the realm of everyday experience, however; in the latter realnl, everything moves fairly slowly and relativistic effects are not manifested. If the speed of light were much smaller, the effects of special relativity would be more prominent and our intuition concerning the nature of time would be quite different.




















No comments